

The sum of all the weighted, shifted copies of g). Weighted and shifted copies of g and, on the right, the integral ( i.e. The bottom pair of graphs shows, on the left, the superposition of several Various values of x and, on the right, that shifted function g multiplied by The next three pairs of graphs show (on the left) the function g shifted by The top pair of graphs shows the original functions.

How you can think about the convolution, as giving a weighted sum of shiftedĬopies of one function: the weights are given by the function value of the secondįunction at the shift vector. Think about this in terms of the illustration below. Note that it doesn't matter which function you take first, i.e. The cross in a circle is used to indicate the convolution operation. Is a function of a new variable u, as shown in the following equations. Taken over the variable x (which may be a 1D or 3D variable), typicallyįrom minus infinity to infinity over all the dimensions. Of one function at x times another function at u-x. Mathematically, a convolution is defined as the integral over all space Proof of the convolution theorem, but it may be more difficult!
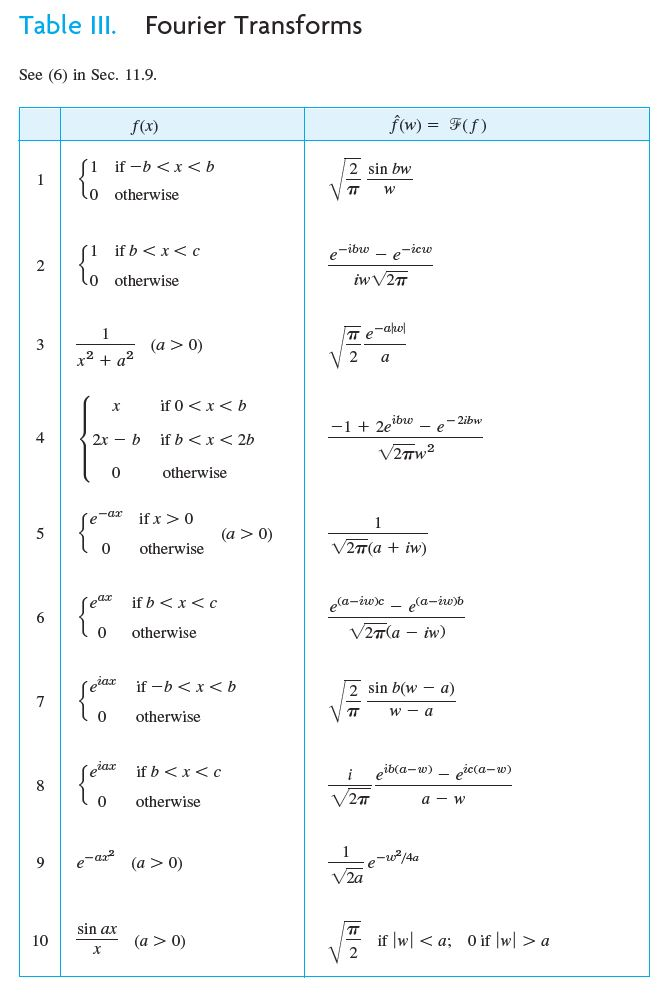
Understanding theĬoncept of a convolution operation is more important than understanding a To as the convolution theorem, it is convenient to carry out calculationsīut first we should define what a convolution is. Because of a mathematical property of the Fourier transform, referred Convolutions arise in many guises, as will be shownīelow. One of the most important concepts in Fourier theory, and in crystallography,


 0 kommentar(er)
0 kommentar(er)
